Víctor González, High School Main Educator
Various academic programs consider geometry as another academic subject. However, for Waldorf pedagogy, geometry is a core part of what it does; it would be very difficult to find any activity within the pedagogy that is not related to geometry, starting from kindergarten to grade 12.

First seven-year term
Antonio Machado says in his immortal poem caminante no hay camino…. Two of the most iconic activities experienced in a preschool are walking in a line and rounds. Although in this seven-year period, the work that the teachers do is focused on exercising the will; It is from imitation that children experience a line (row) and a curve (round). Winding a ribbon necessarily produces a spiral. Thus, children live immersed in geometric concepts without this being the purpose.
Second seven-year term
Class One
Children subtly break into the second seven years and the indicators of maturity set the tone to awaken something that will accompany them for the rest of their lives, they will meet: Miss Curve and Miss Straight Line. The awakening of the so-called creative fantasy allows children to learn through images and that is how the two fundamental strokes appear, in other words, they meet the geometric alphabet that only consists of two elements: lines (straight) and curves. It is with these two elements that the first strokes of the letters and numbers will gradually be incorporated. The magical epic of writing will begin here and will accompany them for the rest of their lives.
Class Two
Many other processes begin in class 2, one of which is manifested in the concept of symmetry. Usually a story tells a simplified landscape of a mountain that is reflected in a lake. Very specifically for Guatemala, that image (a lake with mountains) is very familiar. This is usually the starting point, but it will transform from axial symmetries, which are due to a traditionally red line. Although there have already been signs of which hand will be dominant, it is usually in this period where the dominant use of one hand for writing and other tasks is established.
Class Three
For this degree, a deep and significant theme underlies: metamorphosis. Through images, the aim is to capture the process of transformation from straight lines to curves and vice versa. Although other pedagogies and psychology itself could provide an explanation for doing the above and giving names to the cognitive processes; For Waldorf pedagogy it is of utmost importance that between one image and another, that is, between one step and another, it is the child’s imagination that carries out said transformation, since in this way the process remains alive in him.
Class Four
After the Rubicon, for children, establishing their own limits becomes a constant. Thus, the eurythmic gesture of the E, the cross stitch or Ragnarok are elements according to this process. On the other hand, it is usually here when children draw the iconic Celtic knots. It should be noted that Thomas Wildgruber, Trinus’ mentor, has done a magnificent job compiling pre-Columbian fretwork and motifs that appeal to the same motor, intellectual and emotional qualities as Celtic knots; having in both more tools for children to delve into: up and down, crossing and personal space.
Class Five
For grade 5, freehand geometry appears and begins to appeal to the faculties of thinking through imagination, it is here where a balance can be done to observe a summary of skills of concepts and skills: the straight and curved line from class 1, the symmetry from class 2, the metamorphosis (transformation) from class 3, the complexity and patience of class 4 when carrying out their work, converge in drawings as elaborate, complex and significant as: the two Theorems of Thales, several graphic demonstrations of the Pythagorean Theorem, perpendicular and parallel lines, just to name just a few. It is important to mention that definitions are not the priority, since in this seven-year period beauty is the aspect that must prevail.
Class Six
Geometry with instruments arrives to never leave, from this moment on, children, many of whom must already be considered youngsters, enter a world in which the ruler, the pencil and the compass become the keys to a world full of beauty and precision. It is at this moment where the definitions that were worked on previously take on new meaning. An example would be the simple straight line, which their imagination should be infinite, but on paper has to be limited by two points and called: segment. Traces and more traces, curves that look like straight lines, straight lines that envelop curves, geometric constructions, parallelism and perpendicularity become everyday themes throughout the year. And to top it off: apply color to make it their own. White Magic teacher George Glöckler used to say.
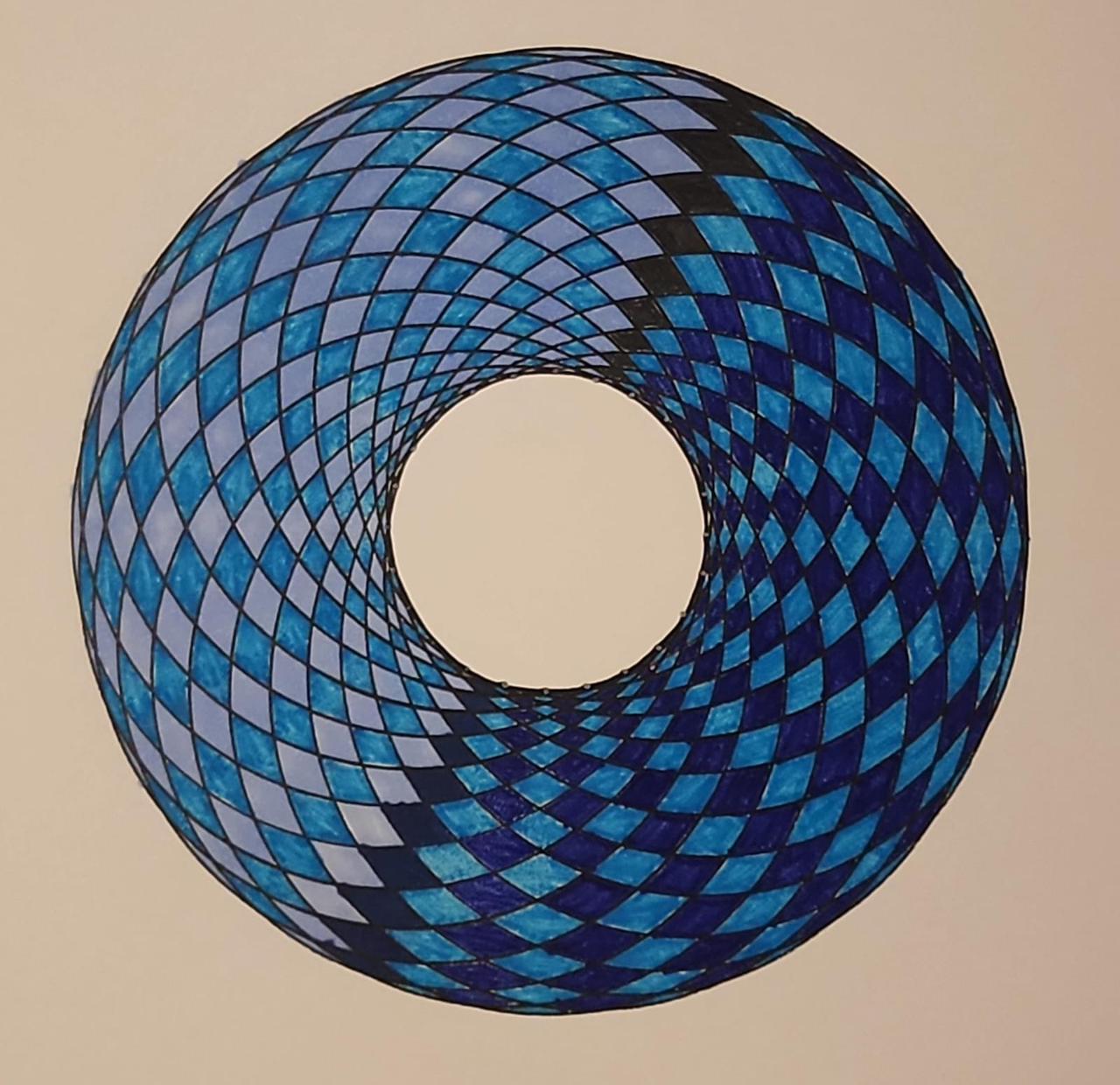
Class Seven
How do you describe the reality of a new world? There are no photographs and painting landscapes as they seem, require more than will. It is then that perspective appears to accompany the explorers. The evolutionary process that youngsters go through is more oriented towards thinking and as such, perspective provides in the geometric and metaphorical sense, the opportunity to interpret reality through laws that at times will be counterintuitive, at times will be obvious and at times will be completely incomprehensible, until grade 9.
Class Eight
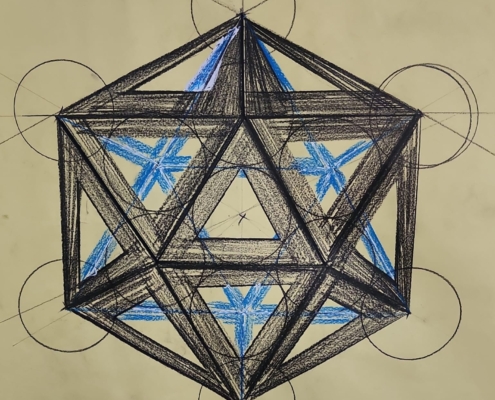
The first seven-year period of education came to an end. Although there are still several years of education for Waldorf pedagogy, it is in this year where it is suggested to make a synthesis, throughout the year, of everything learned in this septenium. In that sense, geometry accompanies this grade through one of the most iconic themes of pedagogy: Platonic solids. Going from 2D drawings to a surface that surrounds a volume makes many concepts take on a new meaning and meaning. The relationship circle/circumference/sphere, area/surface, volume/space, to name a few examples, allows teenagers to relate to their environment in a different way.

Class Nine
The start of High School requires new challenges and concepts. It is then that Projective Geometry bursts in to accompany the revolutions that were seen the previous year and those that are usually seen in this grade. Can an object be seen from three different perspectives simultaneously in a natural setup? The simple answer is no, but it is at this moment where we must begin to accompany teenagers in those uncomfortable questions: What if it could be done, even if it were not natural? What would it look like? What is this going to be useful on a daily basis?. What they see, that is, what they perceive, reality and how to express it will not coincide in most cases, in that sense, projective geometry can be a vehicle for doing, feeling and thinking to converge.
At Trinus we seek to ensure that the education of our children and teenagers has a continuous academic content full of images and living processes, not only in geometry. Since in this way it has been empirically proven that doing so guarantees a high academic standard that has nothing to prove to the so-called traditional pedagogies.